draw a factor tree for the number 24

Factors of 24

Factors of 24 are those numbers that divide 24 completely without leaving any remainder. There are 8 factors of 24 among which 24 is the biggest factor and 2 and 3 are its prime factors. The prime factorization of 24 can be done by multiplying all its prime factors such that the product is 24. Let us learn about all factors of 24, the prime factorization of 24, and the factor tree of 24 in this article.

1.	What are the Factors of 24?
2.	Prime factorization of 24
3.	Factor tree of 24
4.	Factors of 24 in Pairs
5.	FAQs on Factors of 24

What are the Factors of 24?

The factors of 24 can be listed as 1, 2, 3, 4, 6, 8, 12, and 24. According to the definition of factors, the factors of 24 are those numbers that divide 24 without leaving any remainder. In other words, we can say if two numbers are multiplied and the product is 24, then the numbers are the factors of 24. It means that 24 is completely divisible by all these numbers. Apart from these, 24 also has negative factors that can be listed as, -1, -2, -3, -4, -6, -8, -12, and -24. For negative factors, we need to multiply a negative factor by a negative factor, like, (-6) × (-4) = 24.

How to Find the Factors of 24?

Factorization of a number means writing the number as a product of its factors. The most commonly used method to find the factors of a number is using the multiplication method. Let us find the factors of 24 using multiplication.

Factors of 24 using Multiplication Method

Let us find the factors of 24 using the multiplication method using the following steps.

• Step 1: In order to find the factors of 24 using the multiplication method, we need to check what pairs of numbers multiply to get 24. So, we need to divide 24 by natural numbers starting from 1 and go on till 9. We need to make a note of those numbers that divide 24 completely.
• Step 2: The numbers that completely divide 24 are known as its factors. We write that particular number along with its pair and make a list as shown in the figure given above. As we check and list all the numbers up to 9, we automatically get the other pair factor along with it. For example, starting from 1, we write 1 × 24 = 24, and 2 × 12 = 24 and so on. Here, (1, 24) forms the first pair, (2, 12) forms the second pair and the list goes on as shown. So, as we write 1 as the factor of 24, we get the other factor as 24; and as we write 2 as the factor of 24, we get 12 as the other factor. Like this, we get all the factors.
• Step 3: After the list is noted, we get all the factors of 24 starting from 1 up there, coming down and then we go up again up to 24. This gives us a complete list of all the factors of 24 as shown in the figure given above.

Therefore, the factors of 24 can be listed as 1, 2, 3, 4, 6, 8, 12, and 24. Now, let us learn about the prime factorization of 24.

Prime Factorization of 24

Prime factorization is a way of expressing a number as a product of its prime factors. The prime factors of a number are those factors that are prime numbers. The prime factorization of 24 can be done using the following steps. Observe the figure given below to understand the prime factorization of 24.

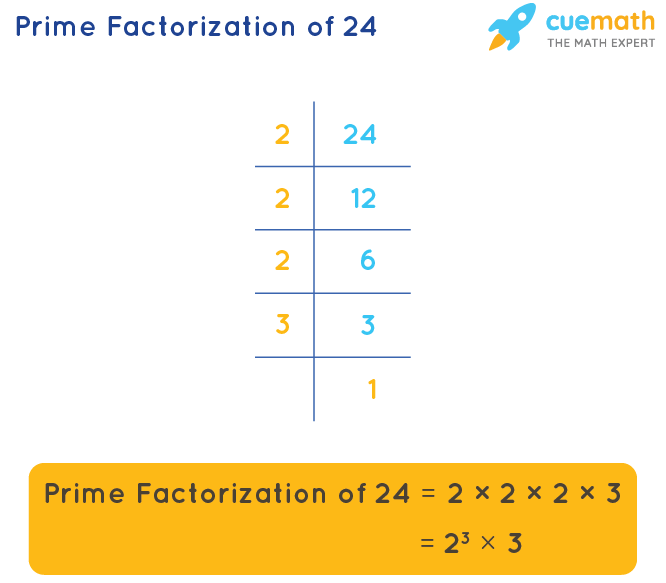
• Step 1: The first step is to divide the number 24 with its smallest prime factor. We know that a prime factor is a prime number which is a factor of the given number. So, with the help of divisibility rules, we find out the smallest factor of the given number. Here, we get 2. Therefore, 2 is the smallest prime factor of 24. So, 24 ÷ 2 = 12
• Step 2: We need to repeatedly divide the quotient by 2 until we get a number that is no more divisible by 2. So, we divide 12 again by 2 which is 12 ÷ 2 = 6
• Step 3: Divide 6 again by 2 which results in 6 ÷ 2 = 3
• Step 4: Divide the quotient by 3 again which is 3 ÷ 3 = 1
• Step 5: We need not proceed further as we have obtained 1 as our quotient.
• Step 6: Therefore, the prime factorization of 24 is expressed as 2 × 2 × 2 × 3 = 2³ × 3; where 2 and 3 are prime numbers and the prime factors of 24.

Factor Tree of 24

We can also find the prime factors of 24 using a factor tree. The factor tree of 24 can be drawn by factorizing 24 until we reach its prime factors. These factors are split and written in the form of the branches of a tree. The final factors are circled and are considered to be the prime factors of the 24. Let us find the prime factors of 24 using the following steps and the factor tree given below.

• Step 1: Split 24 into two factors. Let us take 2 and 12.
• Step 2: Observe these factors to see if they are prime or not.
• Step 3: Since 2 is a prime number we circle it as one of the prime factors of 24. We move on to 12, which is a composite number and further split it into more factors. In other words, we repeat the process of factorizing 12 and splitting it into branches until we reach a prime number.
• Step 4: Here, we get 2 and 6. So, we circle 2 because it is a prime number and we split 6 into 2 and 3. At this stage, we are left with prime numbers, 2 and 3. We circle them since we know that they cannot be factorized further. This is the end of the factor tree.
• Step 5: Therefore, the prime factors of 24 = 2 × 2 × 2 × 3

Note: It should be noted that there can be different factor trees of 24. For example, we can start by splitting 24 into 4 and 6. Then, 4 can be split further into 2 and 2, while 6 can be split into 2 and 3. Finally, we can observe the same prime factors, that is, 24 = 2 × 2 × 2 × 3

Factors of 24 in Pairs

The factors of 24 can be written in pairs. This means that the product of the pair factors of 24 is always 24. The factors of 24 in pairs can be written as shown in the table given below:

Factors	Positive Pair Factors
1 × 24 = 24	1, 24
2 × 12 = 24	2, 12
3 × 8 = 24	3, 8
4 × 6 = 24	4, 6

It is possible to have negative pair factors as well because the product of two negative numbers also gives a positive number. Let us have a look at the negative pair factors of 24.

Factors	Negative Pair Factors
-1 × -24 = 24	-1, -24
-2 × -12 = 24	-2, -12
-3 × -8 = 24	-3, -8
-4 × -6 = 24	-4, -6

The following points explain some features of the pair factors of 24.

• The pair factors of the number 24 are whole numbers in pairs that are multiplied to get the original number, i.e., 24.
• Pair factors could be either positive or negative but they cannot be fractions or decimal numbers.
• The positive pair factors of 24 are as follows: (1, 24), (2, 12), (3, 8), and (4, 6). The negative pair factors of 24 are (-1, -24), (-2, -12), (-3, -8), and (-4, -6).

Important Notes

• Only composite numbers can have more than two factors. Since 24 is a composite number, it has more than two factors.
• Every factor of a given number is either less than or equal to the given number.
• The number of factors of a given number is finite. 24 has 8 factors.
• Factors of 24 are those numbers that divide 24 completely without leaving any remainder.
• 24 has a total of 8 factors: 1, 2, 3, 4, 6, 8, 12, and 24.
• There is a trick to calculate the total number of factors of a number. For example, 24 = 2 × 2 × 2 × 3 = 2³ × 3. We get the prime factorizations of 24 as 2³ × 3. Just add one (1) to the exponents 3 and 1 individually and multiply their sums. (3 + 1) × (1 + 1) = 4 × 2 = 8. This means 24 has 8 factors in all.

Points to remember

Let us recollect the list of the factors, the negative factors, and the prime factors of 24.

• Factors of 24: 1, 2, 3, 4, 6, 8, 12 and 24
• Negative Factors of 24: -1, -2, -3, -4, -6, -8, -12 and -24
• Prime Factors of 24: 2, 3
• Prime Factorization of 24: 2 × 2 × 2 × 3 = 2³ × 3
• Sum of Factors of 24: 60

☛ Related Articles

• Factors of 224 - The factors of 224 are 1, 2, 4, 7, 8, 14, 16, 28, 32, 56, 112, 224
• Factors of 243 - The factors of 243 are 1, 3, 9, 27, 81, 243
• Factors of 245 - The factors of 245 are 1, 5, 7, 35, 49, 245
• Factors of 21 - The factors of 21 are 1, 3, 7, 21
• Factors of 42 - The factors of 42 are 1, 2, 3, 6, 7, 14, 21, 42
• Factors of 48 - The factors of 1, 2, 3, 4, 6, 8, 12, 16, 24, 48

Examples on Factors of 24

1. Example 1: Write all the positive factors of 24.

   Solution:

   All the positive factors of 24 are 1, 2, 3, 4, 6, 8, 12 and 24.

2. Example 2:

   State true or false with respect to the factors of 24.

   a.) 9 and 6 are factors of 24.

   b.) 2 and 3 are the prime factors of 24.

   Solution:

   a.) False, 6 is a factor of 24 but 9 is not a factor of 24.

   b.) True, 2 and 3 are the prime factors of 24.

3. Example 3: List the positive and negative pair factors of 24.

   Solution:

   The positive pair factors of 24 are as follows: (1, 24), (2, 12), (3, 8), and (4, 6). The negative pair factors of 24 are (-1, -24), (-2, -12), (-3, -8), and (-4, -6).

go to slidego to slidego to slide

Explore amazing ways to learn all about factors!

Let our top math educators solve in steps that you follow. Grasp the core logic and never struggle with factors again.

Book a Free Trial Class

Practice Questions on Factors of 24

go to slidego to slide

FAQs on Factors of 24

What are the Factors of 24?

The factors of 24 are 1, 2, 3, 4, 6, 8, 12, 24 and its negative factors are -1, -2, -3, -4, -6, -8, -12, -24.

What are the Prime Factors of 24?

The prime factors of 24 are 2 and 3. The prime factors of a number are those factors that are prime numbers. In this case, if we do the prime factorization of 24, we get 2 × 2 × 2 × 3 = 2³ × 3, where 2 and 3 are prime numbers and the prime factors of 24.

What are the Common Factors of 24 and 32?

The factors of 24 can be listed as 1, 2, 3, 4, 6, 8, 12, and 24. the factors of 32 can be listed as 1, 2, 4, 8, 16, and 32. Among these, we can list the common factors of 24 and 32 as 1, 2, 4, and 8. Now, we can find the Greatest Common Factor (GCF) of 24 and 32 which is 8.

What is the Greatest Common Factor of 24 and 23?

The factors of 24 are 1, 2, 3, 4, 6, 8, 12, 24 and the factors of 23 are 1, 23. So, we can see that 24 and 23 have only one common factor which is 1. Hence, the Greatest Common Factor (GCF) of 24 and 23 is 1. This also implies that 24 and 23 are co-prime numbers.

What are the Common Factors of 24 and 21?

The factors of 24 are 1, 2, 3, 4, 6, 8, 12, 24 and the factors of 21 are 1, 3, 7, and 21. Hence, (1, 3) are the common factors of 24 and 21.

What is the Sum of the Factors of 24?

The sum of all the factors of 24 can be calculated by adding 1, 2, 3, 4, 6, 8, 12, 24 which is 1 + 2 + 3 + 4 + 6 + 8 + 12 + 24 = 60.

baileygliver1994.blogspot.com

Source: https://www.cuemath.com/numbers/factors-of-24/

Share this post